Celebrating Diverse Mathematics

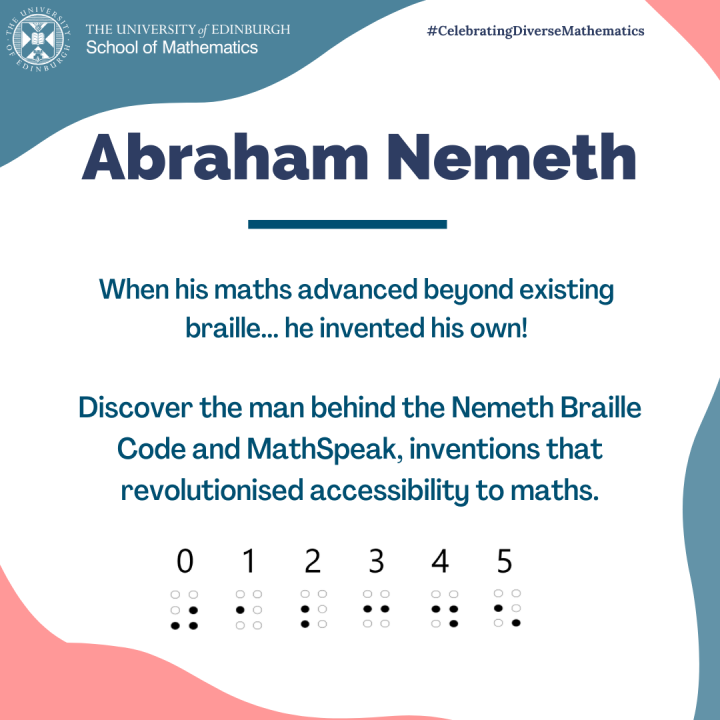
Abraham Nemeth (1918-2013) was born in New York to a large Hungarian-Jewish family, and was born blind as a result of a genetic disorder. Attending Brooklyn College, he first studied mathematics and physics, before going on to earn a PhD in mathematics from Wayne State University.

As his mathematics became more advanced, Nemeth found he needed a braille code that was better suited for handling the kinds of mathematical data he was handling, and so in 1952 he published the Nemeth Braille Code for Mathematics and Science Notation, which is still in use today.

He was also the creator of a system of verbally communicating mathematical text called MathSpeak, which he used to both read maths text aloud and have spoken maths material transcribed accurately. 

Alan Turing (1912-1954) is widely considered to be the father of theoretical computer science and artificial intelligence, as he created the Turing machine which laid the foundations for the general-purpose computer we all know today.

Born in London, Turing graduated at King’s College Cambridge with a degree in mathematics, and would go on to earn a PhD from Princeton. During the Second World War, Turing worked for the UK government at Bletchley Park, the centre of Britain’s codebreaking efforts. Here he played a crucial role in cracking intercepted coded messages that enabled the Allies to defeat the Axis powers in many crucial engagements of the war.

Despite his heroic contributions during the war, Turing was prosecuted for “homosexual acts” and was forced to undergo chemical castration until his death in 1954. It wasn’t until 2009 that the UK Government issued an official apology for this, and today Turing remains one of the most famous mathematicians in history. 

Image credit: https://commons.wikimedia.org/wiki/File:Alan_Turing_Age_16_Colorized.jpg  

Aryabhata (c. 476-550 CE) was born in India and is famous for his approximation of pi to 4 decimal places.

In his texts, Aryabhata discusses how pi ‘approaches’ the circumference of a circle. Some translators and commentators have suggested that this indicates Aryabhata’s perception of pi as unmeasurable.

This is the first account which could potentially indicate knowledge of the irrationality of pi, something which wasn’t considered in Europe until 1761. 

Bernard Morin (1931-2018) was a French mathematician who had lost his sight as a child. He earnt his PhD from the Centre National de la Recherche Scientifique and went into the field of topology.

He is known for discovering the Morin Surface (see image), a half-way model for the sphere eversion, which he used to prove a lower bound on the number of steps required to turn a sphere inside out.

Morin was also a member of the group that first demonstrated an eversion of the sphere, and would himself discover the first parametrisation of Boy’s surface. 

Image credit: File:MorinSurfaceCrossView.PNG - Wikipedia

Brahmagupta (c.598-668 CE) was an Indian mathematician who was the first to give meaning to zero. Before Brahmagupta, Europeans had considered zero as merely a symbol representing a lack of quantity. Brahmagupta changed this definition and began to consider zero as a number.

Another accomplishment of Brahmagupta’s was the Brahmagupta-Fibonacci identity, an important identity in algebra. The identity was initially proved by Diophantus of Alexandria, however Brahmagupta proved a more general case around three centuries later. The identity was introduced to Europe by Fibonacci some 600 years after Brahmagupta proved it, after which it was typically credited to Fibonacci, not Brahmagupta. 

Brahmagupta also devised an exact formula to calculate the area of cyclic quadrilaterals, as seen in the image.

Elbert Frank Cox (1895-1969) was the first African American to get a PhD in mathematics, and most likely the first black person ever. Cox most notably worked on specialised polynomials and how they could be used to solve differential equations.

Cox studied at Cornell University and endured many difficulties whilst there, as the Ku Klux Klan were very active in his area. Elbert Frank Cox obtained his PhD in 1925 before moving to Howard University to teach. Despite Cox’s credentials and abilities, he was outranked by many other professors, and was never given the credit he deserved for his work whilst at university. This was largely because several universities refused to consider his thesis or publish any of his papers.

Eventually Cox got two papers published, on Euler polynomials and systems of grading. Cox was very active in teaching, and his students performed very well. He helped with the creation of Howard University’s PhD programme and paved the way for other mathematicians of colour. 

Emmy Noether (1882-1935) was a German mathematician who was born to a Jewish family. Her work was centred around abstract algebra and mathematical physics, although it’s said she allowed her colleagues and students to use her ideas without credit, to help develop their careers, so there is no way of knowing the full extent of her contributions.

Noether developed some of the most important innovations of 20th century mathematics and completely revolutionised the field of algebra, with her development of ring theory and the theory of ideals. However, despite her many achievements she was never elected to the Academy of Sciences in Germany and was not promoted to the position of a full professor.

Noether’s right to teach was then withdrawn completely when the Hitler’s administration took charge of Germany, as they didn’t want so-called ‘Jewish mathematics’ being taught. Noether died of complications in a surgery 4 years before the Second World War broke out, and whilst her work is widely used in algebra, she is still not given the credit she deserved. 

Hypatia (c. 350–415 AD) was a Roman mathematician who wrote a commentary on the famous mathematical text Arithmetica, by Diophantus. Her commentary was so good, it has now been incorporated into the text itself and is almost indistinguishable.

There is no surviving evidence of any independent works of mathematics, however this was common of Roman times, where most scholars debated and commented on other existing works.

Most of Hypatia’s contributions to mathematics were preserving important mathematical books and adding vital commentary to them, which made them accessible for younger scholars to learn from. This allowed the mathematical study from before her time to progress through history, carried on by other scholars understanding of Hypatia’s commentary. 

Jamshīd al-Kāshī (c. 1380-1429) was a Persian mathematician, born in modern day Iran. His work remains mostly unknown to Europe.  

Al- Kāshī was the first to conceive the law of cosines and his approximation of sin(1) was unsurpassed until the sixteenth century. He developed an iterative method for solving cubic equations, which wasn’t discovered in Europe for many more centuries, and devised a method similar to Newton’s method, around 300 years before Newton.  

Al-Kāshī also made contributions to the exploration of binomial coefficients, building on the work of the Persian polymath Omar Khayyam. Pascal’s triangle is known as Khayyam’s triangle in Persia, as the properties of the triangle and binomial coefficients were discovered by Persians long before Blaise Pascal explored them. 

Perhaps best known from his depiction in the book and film “A Beautiful Mind”, John Forbes Nash Jr. (1928-2015) was an American mathematician who made groundbreaking contributions to game theory, differential geometry, and the study of partial differential equations, all whilst living with schizophrenia.

Born on June 13, 1928, in Bluefield, West Virginia, his theories came to be vastly important to economics and he would share the Nobel Memorial Prize in Economic Sciences with fellow game theorists Reinhard Selten and John Harsanyi in 1994.

Nash was admitted to hospital several times in his life due to poor mental health, but he would nonetheless continue to offer major contributions to mathematical studies, including developing the Nash embedding theorem for algebraic geometry, and an award-winning PhD on non-cooperation in game theory. 

Image credit: https://quotepark.com/authors/john-nash/  

Katherine Johnson (1918-2020) was an African American mathematician who worked as a 'computer' for NASA. She did the orbital calculations which ensured the success of the first US crewed space flight and many later orbital and space flights. 

Johnson faced many difficulties in her life and career path, as she was discriminated against based on her race and her gender, and the intersection of these. She was one of the first black students to be integrated into a majority white college and would have experienced many prejudices whilst there.  

Johnson was awarded the Presidential Medal of Freedom by Barack Obama in 2015. There was also a film made in 2016, called Hidden Figures, which documents her life and the lives of many black women in NASA, who were vital to the success of many space missions and whose contributions have largely been forgotten. 

Leonhard Euler (1707-1783) was a Swiss mathematician who founded graph theory and topology, and made significant contributions to analytic number theory and complex analysis. He gave the current definition of the mathematical constant 'e', or Euler's number, as it was named. This constant is the base of the natural logarithm and is widely used throughout the world. 

Throughout Euler's career, his eyesight deteriorated, until he was almost fully blind by the age of 59. However, with the aid of scribes, it is said Euler still produced a mathematical paper every week, even once his vision had totally gone. 

Euler made some of the most famous and important contributions to mathematics, and is rightfully credited for his work, with many of his discoveries named after him. One of these is Euler's identity, also known as the perfect example of mathematical beauty. The identity connects five of the most fundamental quantities in mathematics; pi, i (the imaginary unit, or the square root of -1), e, 1, and 0. 

Known best for her work with Lockheed Corporation and their designs for air and spacecraft, Mary Golda Ross (1908-2008) was the first known Native American female engineer and mathematician.

She earned her first degree at 20 years old and was hired by Lockheed Corporation to solve design issues with their high speed flightcraft. Ross then went on to work on many projects for Lockheed, designing concepts for interplanetary space travel, earth-orbiting flights, and early studies into orbiting satellites.

Even after her retirement she worked tirelessly recruit young women and Native American youth into STEM careers, and was a staunch supporter of the American Indians in Science and Engineering Society (AISES) and the Council of Energy Resource Tribes. 

Sofya Kovalevskaya (1850-1891) was the first woman to obtain a doctorate in mathematics and the first woman appointed a full professorship in north Europe. Kovalevskaya was tutored by Karl Weierstrass, since as a woman she was not allowed into classes, not even to audit them. 

One of her most notable contributions was the ‘Kovalevskaya top’, a highly important discovery into the mathematics of spinning tops. Kovalevskaya also worked with Cauchy and developed the Cauchy-Kovalevskaya theorem, a key theorem in the field of partial differential equations.

She was massively influential in the field of mathematics and had to overcome many obstacles to even begin to study the subject. 

Image credit: https://commons.wikimedia.org/wiki/File:Sofja_Wassiljewna_Kowalewskaja_1_%28Remini_enhanced%29.jpg  

Solomon Lefschetz (1884-1972) was an American mathematician famous for developing the topological aspects of algebraic geometry. A double amputee since an accident that lost him both hands, Lefschetz left a career in engineering to pursue pure mathematics at Clark University in Massachusetts.

He gained a PhD with a dissertation on algebraic geometry that would open the door to his academic career and creation of the field of algebraic topology, where he would revolutionise combining algebraic concepts with the study of three-dimensional shapes and spaces.

He taught at Princeton for most of his career, where he further contributed to the studies of differential equations, control theory and nonlinear mechanics, and in 1964 he was awarded the National Medal of Science for his contributions. 

Sophie Germain (1776-1831) was a French mathematician who made vital contributions to mathematics. This was despite her being forced to study at night by candlelight, as her parents believed studying mathematics was too difficult for women and would make her go insane.  

However, Germain persisted and obtained lecture notes under another male student’s name. Her mathematical ability was so advanced that a faculty member, Lagrange, requested a meeting with her. He was suspicious because Germain’s work was far superior to that submitted previously by the male student she was appropriating. Lagrange discovered her true identity, but nonetheless continued to tutor her.  

Germain made many substantial contributions to mathematics in her lifetime. Her work on elasticity and the theorems she developed helped build the Eiffel Tower. She was also the first person in 200 years to make progress on Fermat’s Last Theorem, and her work was vital to Andrew Wiles’ proof of the theorem in the late 20th century.  

Sophie Germain was an exceptional mathematician, only more so because of what she overcame to study the subject. Her work is honoured today in many forms, with her development of Sophie Germain primes recognised in the field of number theory. However, her name is not mentioned nearly enough, considering the scope of her dedication and improvement to mathematics as a whole. 

Born in Madras, India, Srinivasa Ramanujan (1887-1920) had next to no formal training in pure mathematics yet became well-known for his contributions to number theory, infinite series, mathematical analysis, and continued fractions.

He compiled almost 4000 results across his life, primarily equations and identities, many of which had never been seen before and included the Ramanujan prime, the Ramanujan theta function, and many more which opened previously unexplored areas of study.

He became only the second Indian member and one of the youngest Fellows of the Royal Society, as well as the first Indian to be elected a Fellow of Trinity College Cambridge. 

In an era when customs typically barred women from education altogether, Wang Zhenyi ( 1768–1797) became an accomplished mathematician and scholar and published her book “The Simple Principles of Calculations” at only 24 years old.

Wang was born to a family of scholars who supported her learning, and with their help she taught herself astronomy, geography, medicine and maths, before deciding to focus on the latter. Conducting experiments in her home, Wang sought to find formulae and theorems to explain the lunar cycle, and became a highly-respected teacher in both maths and science. It was in her role as educator that she revised the works of mathematicians she had learnt from herself – simplifying many of their proofs in the process- and compiled her book.

Alongside mathematics, Wang Zhenyi made significant contributions to astronomy and literature, with her poems gaining her as much fame as her mathematics achievements. 

Image credit: https://www.geograph.org.uk/photo/6863833  

Fibonacci was a famous Italian mathematician, known for the Fibonacci sequence (0, 1, 1, 2, 3, 5, 8, 13, etc.). He studied in North Africa, and it’s highly likely that he discovered the Fibonacci sequence there. Evidence exists showing the use of the Fibonacci sequence in the construction of the Temple of Karnak, Egypt. The sequence was also used in patterning Kente cloth, in Ghana. There is also early existence of this sequence in Sanskrit poetry, found by examining how different kinds of poems can be made with long and short vowels. This work can be traced as far back as 200/300 BC.

The Fibonacci sequence is very closely linked with the Golden Ratio, a dividing property where a square divides into smaller and smaller squares, and the lengths of all the smaller squares add up to be the same length as the original square.

The Golden Ratio was often attributed to the Greeks, although the Greeks never applied the ratio. African villages, however, can be seen to use the ratio in practice, spiralling out from a centre mirroring the Golden Ratio. Evidence of supposed ‘European mathematics’ can be seen everywhere across the world, long before it was written down in Europe.  

It has been long been thought that calculus as we know it today originates from the works of Isaac Newton and Gottfried Leibnitz in the 17th century. But this is not the whole story.

Documents and sources from the Kerala School in southern India show works produced centuries prior to Newton and Leibnitz and using many calculus related concepts such as summation, rate of change, and interpolation.

This evidence suggests that Indian mathematicians were developing and using the basics of calculus around 300 years before its recognised birth in Europe.